Trigonometric Functions of Any Angle
To evaluate a trig function-
(Using Figure Below)
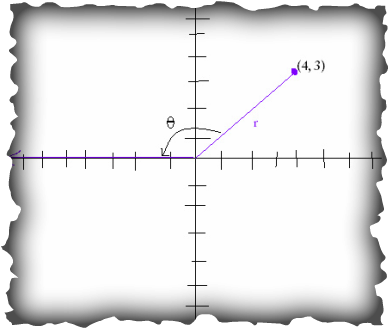
When given a diagram and asked to find trig functions, the formula r= √(x²+y²)
will be used. By finding r (the hypotenuse of the imaginary triangle in Quadrant 1), we can find the trig funtions. To solve for r, simply plug in the x and y values from the point into the equation. We now have r= √(4²+3²). Next, we solve the square roots within the parentheses, which leaves us with r= √(16+9). Add the numbers within parentheses together, which, in this case, gives us r= √(25). Finally, we can solve for y by taking the square root, which equals 5. Now we can find whatever trig function we need, knowing that sine=y/r, cosine=x/r, and tangent=y/x. For this diagram, sine will equal 3/5, cosine will equal 4/5, and tangent will equal 3/4.
When given a diagram and asked to find trig functions, the formula r= √(x²+y²)
will be used. By finding r (the hypotenuse of the imaginary triangle in Quadrant 1), we can find the trig funtions. To solve for r, simply plug in the x and y values from the point into the equation. We now have r= √(4²+3²). Next, we solve the square roots within the parentheses, which leaves us with r= √(16+9). Add the numbers within parentheses together, which, in this case, gives us r= √(25). Finally, we can solve for y by taking the square root, which equals 5. Now we can find whatever trig function we need, knowing that sine=y/r, cosine=x/r, and tangent=y/x. For this diagram, sine will equal 3/5, cosine will equal 4/5, and tangent will equal 3/4.
Evaluating Trig Functions (Continued)
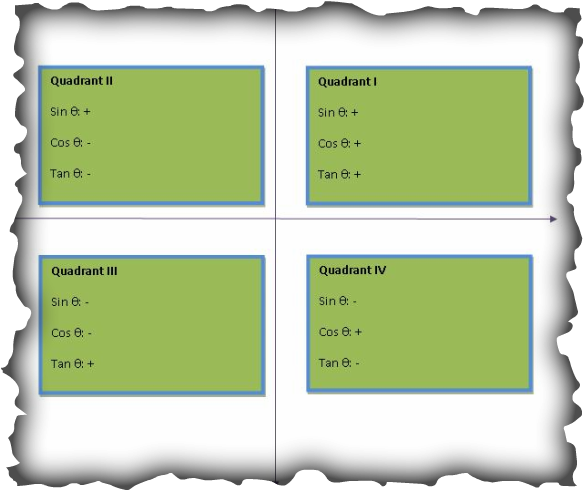
In many cases, you will be given a trig function and expected to find the remaining functions. By knowing which quadrants sine, cosine, and tangent are negative and positive in, you can solve for either x, y, or r and find the other trig functions. Let's say that the problem says that tangent=7/5, and cos>0. Using this information, we can figure out that the triangle is located in Quadrant 1, as that is the only quadrant where both tangent and cosine are positive. Using our equation for r (r= √(x²+y²)), we can plug in the values from tangent and solve. Tangent equals y/x, so the equation for r will be √(5²+7²). Squared, we get √(25+49), which equals √(74). Using our calculator, we find r to be 8.602325267. Now, we can find sine and cosine. Sine=y/r, so sine=7/8.60, or 0.81. Cos=x/r, so cos=5/8.60, or 0.58. You may also leave r in its square root form and simply put the problem into your calculator that way, which should still get you the same answer. The following chart will help you to remember where sine, cosine, and tangent are negative and positive.
Reference Angles
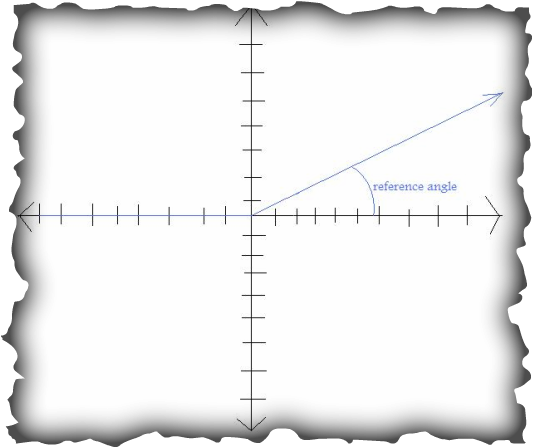
A reference angle is the shortest distance from the terminal side of an angle to the x-axis. Reference angles can be used to find the trig functions of angles greater than 90°.
Finding Reference Angles
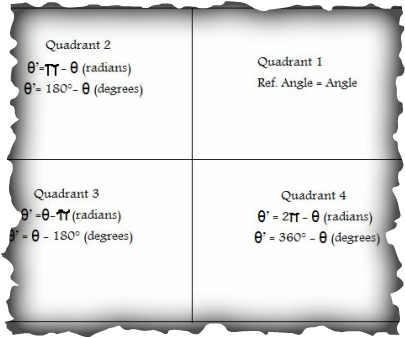
To find a reference angle, simply use the chart above.
For example, if we are told to find the reference angle of 200°, we first use what we know about the unit circle to figure out what quadrant the terminal side of the angle is in. In this case, the terminal side falls in quadrant 3. If we are trying to find the reference angle in radians, then we first must convert the degrees of the angle to radians. This will equal 10π/9. We then look at the equation for radians in the chart above and plug in 10π/9 for θ. The equation will be θ = 10π/9 - π. We will do the same to find the reference angle in degree, except instead of converting to radians, we can simply plug 200° in for θ. The equation will be θ = 200° - 180°.
For example, if we are told to find the reference angle of 200°, we first use what we know about the unit circle to figure out what quadrant the terminal side of the angle is in. In this case, the terminal side falls in quadrant 3. If we are trying to find the reference angle in radians, then we first must convert the degrees of the angle to radians. This will equal 10π/9. We then look at the equation for radians in the chart above and plug in 10π/9 for θ. The equation will be θ = 10π/9 - π. We will do the same to find the reference angle in degree, except instead of converting to radians, we can simply plug 200° in for θ. The equation will be θ = 200° - 180°.
Using Reference Angles to find Trig Functions of Obtuse Angles
We can also use reference angles to find trig functions of obtuse angles. To do this, simply use the corresponding formula above to find the reference angle of the larger angle. Then, looking at the unit circle, we can find the trig function of the reference angle (which is the same function as the larger angle). For example, if we are asked to find the tangent of 120°, we can use the formula θ’=180°-θ to find the reference angle. 180°-120° equals 60°, which is a value found on the unit circle. The tangent of 60° is √3/3, and since we know tangent is negative in quadrant 2, the tangent of 120° will equal -√3/3.